One of the OTHER things I love about MathsJam is that I always come away with a notebook full of new ideas for posts. Most of them are indecipherable, but some stick in the mind. This one is based on a real-life dilemma posed by a friend of Elizabeth, which I’ve depoliticised slightly:

There’s an election to an influential board of scientists. There are four candidates: a pure mathematician (P), an applied mathematician (A), a statistician (S) and (shudder) a chemist (C). You may vote for up to three of the candidates; the two with the most votes will be elected.

Obviously, you want the two mathematicians (P and A) to get in, but you’d rather have the statistician (S) than the chemist (C). Should you vote just for the mathematicians, or for the statistician as well?

With this sort of problem, I tend to start with a simple case and see if I can develop it from there. For example, I’d imagine an electorate of four: a mathematician (you), who prefers P and A, but would accept S; a moderate mathematician who likes A and S but would accept P; a moderate chemist who likes A and S but would accept C; and a chemist who likes C and S but would accept A. Got that? 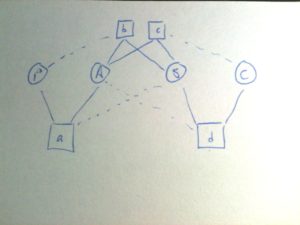Candidates and electorate If everyone votes for their two preferred candidates, we end up with one vote each for P and C, while A and S get three apiece and get elected (in fact, this is an acceptable outcome for everyone: everyone has at least one of their favourites and no-one hates any of the candidates).

Could anyone swing the vote from an OK result to a good one? Actually, no: the only people who aren’t fully happy are the extremists (you and the chemist) - and you’ve both already voted for your preferred candidates. The moderates are quite content, thank you: they’ve no reason to vote for either extremist and risk one of their preferred candidates losing.

In this model, it turns out, the moderates always win: let’s say you have $a$ voters like you (P, A and maybe S), $b$ voters in the A, S and maybe P camp, $c$ who like A, S and maybe C, and $d$ who like S, C and maybe A.

In that case, the candidates get the following numbers of votes:

P: $a$ A: $a + b + c$ S: $b + c + d$ C: $d$

In particular, unless there are no moderates at all, A always beats P and S always beats C. The only way an extremist can beat a moderate is if there is a majority of extremists - and whether your pet extreme candidate is winning or losing, it doesn’t make sense to help the candidate you don’t want to win.

Unless, as I said, there are no moderates. In an evenly polarised contest ($a=d>0$ and $b=c=0$), you end up with a tie between all four candidates. In that situation, voting additionally for your least-hated candidate would make sense. This, though, is the only situation I can come up with (under this model) where you would have any reason to express a preference between your two disliked candidates.

Even if you’re a moderate, there’s no circumstance in which using your third vote takes you from a poor outcome to a better one - you’d only ever use it to express a preference between the two extreme candidates, and there’s no situation where both extreme candidates are in the running.

Arrow’s Theorem says there’s no perfect voting system, but I gather preference voting is generally found to be a system that’s easy to understand and that feels fair - even if, in this election, it leads to the election of a statistician.

As Elizabeth’s friend says, you should never vote for someone you don’t want to get in: this model seems to support that excellent guiding principle.

- Thanks to Elizabeth for the puzzle, and to Alisdair, Rob and Francis for the discussion.